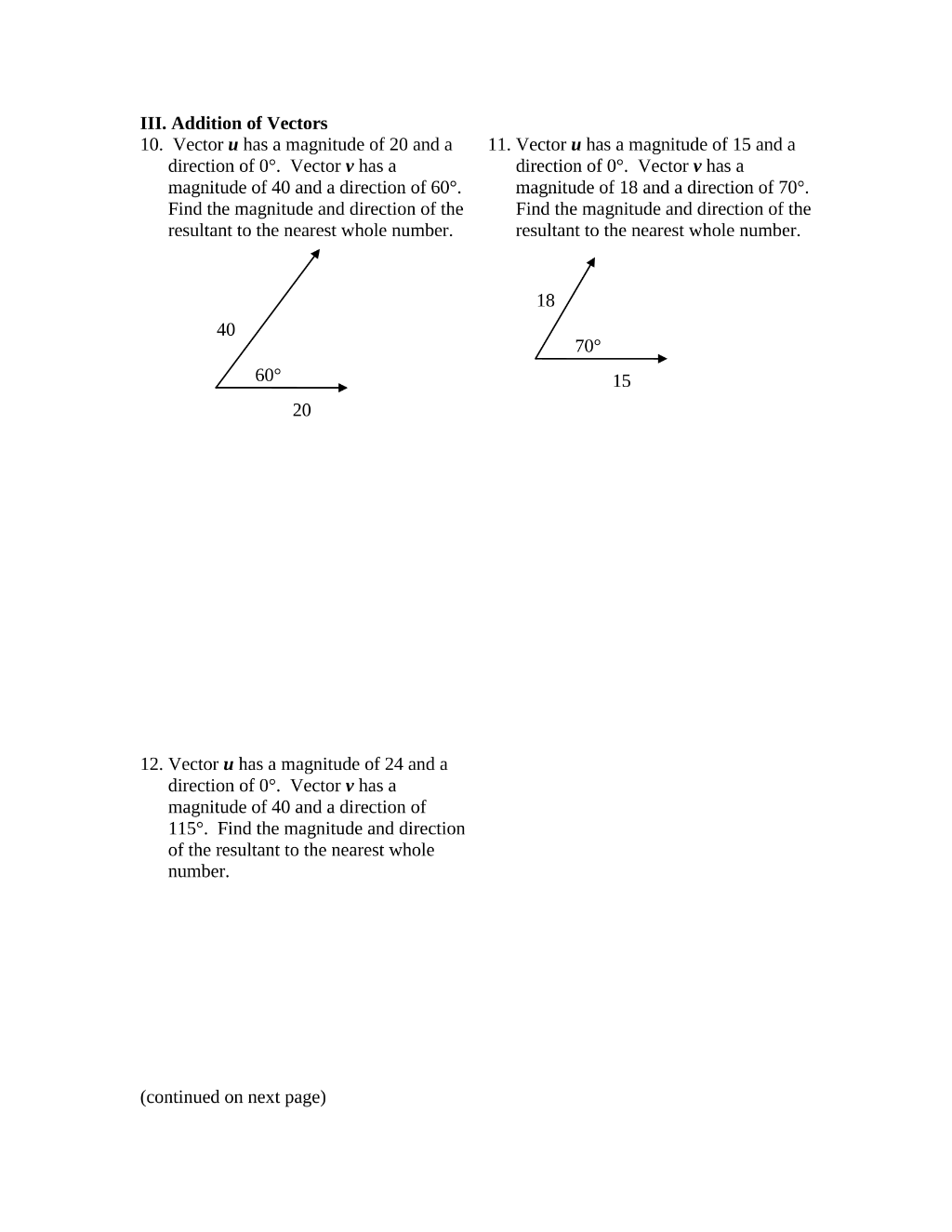
Preview 1

Let H be the quaternion algebra. Let x = a+bi+cj+dk ∈ H. Define its conjugate to be the quaternion x = a − bi− cj −dk. (i) Show that xx = xx = (a2 +b2 + c2 + d2). (ii) Show that H is a division algebra, i.e., xy = 0 =⇒ x = 0 or y = 0. (ii) Let q be a nonzero quaternion. Show that {q, qi, qj, qk} is a basis of H.
Preview 1

Preview 2
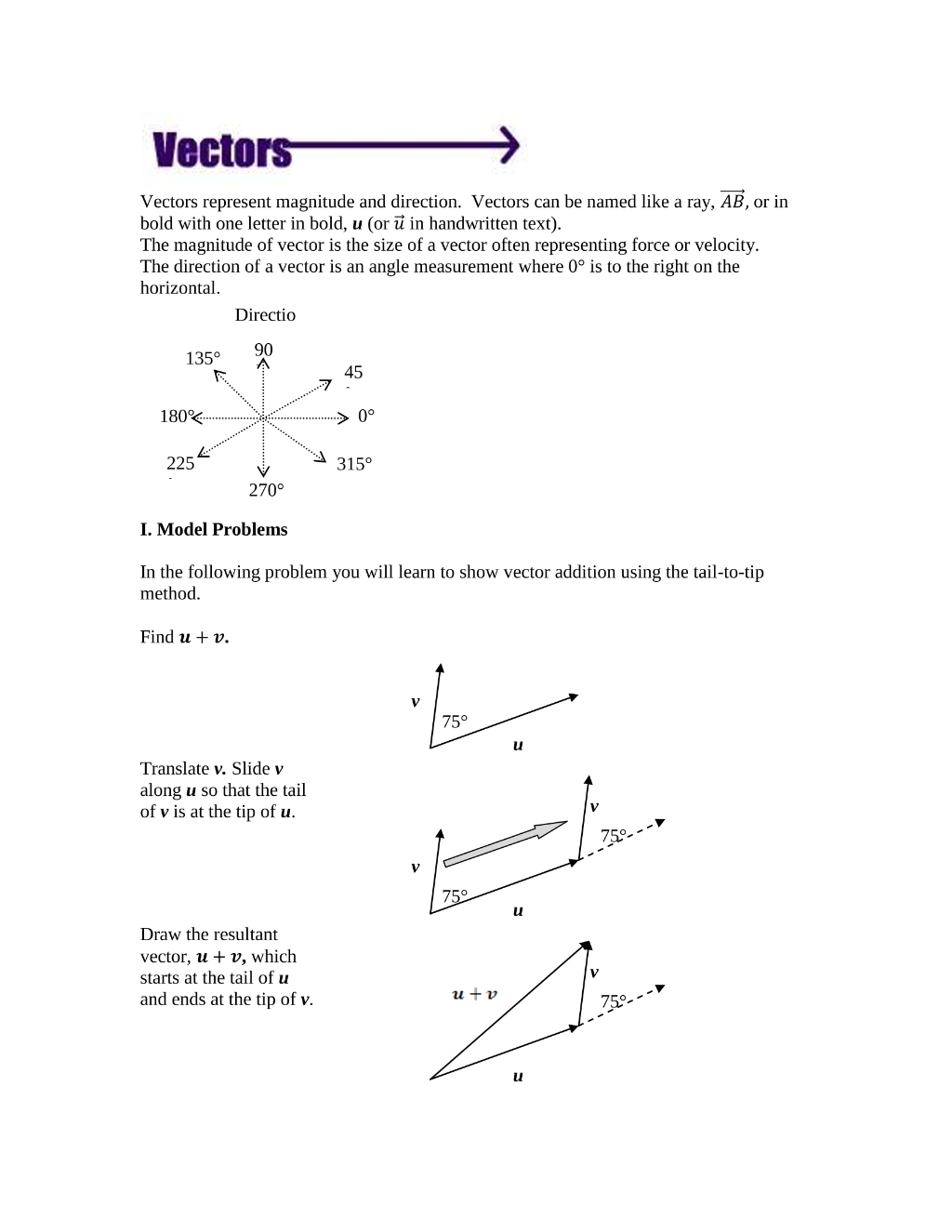
Preview 3
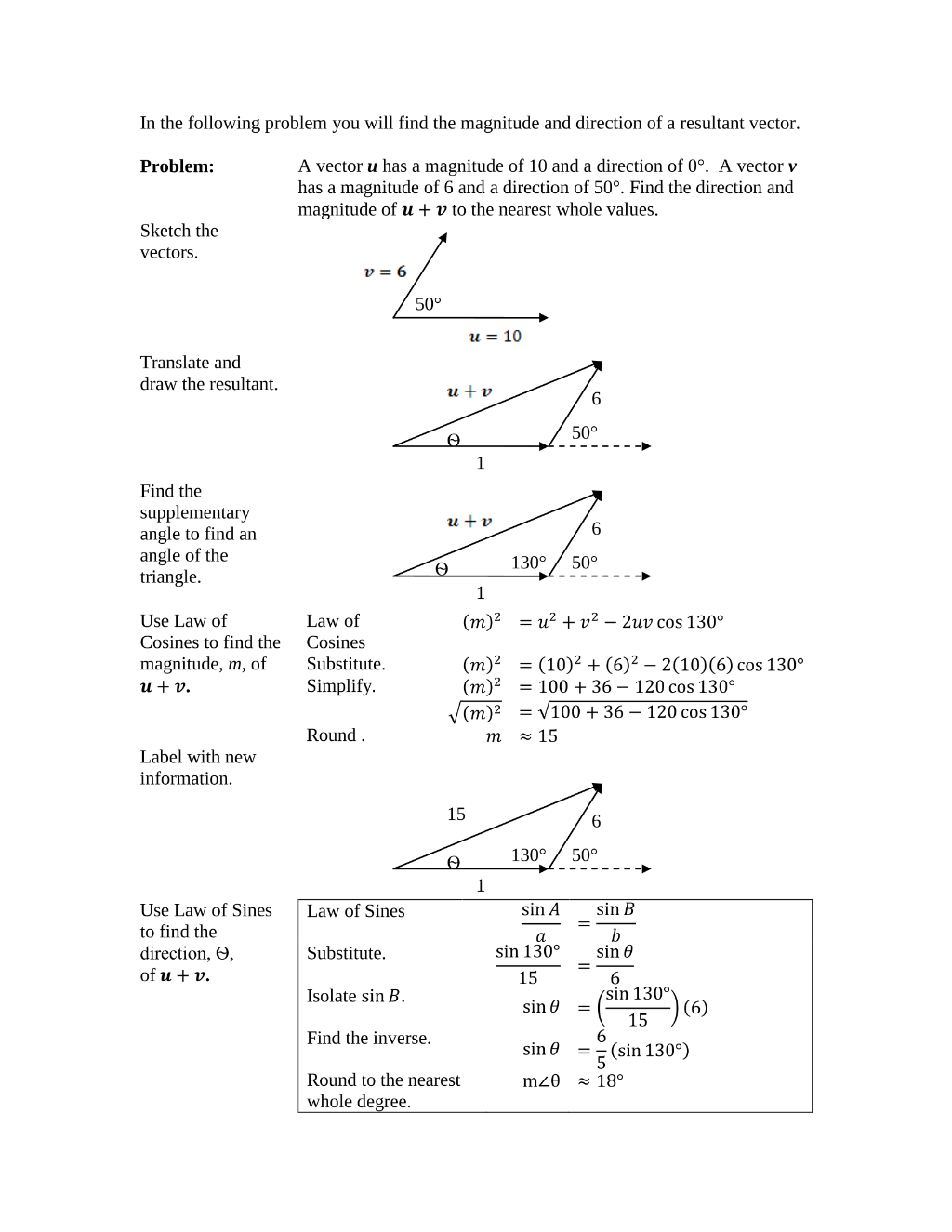
Preview 4
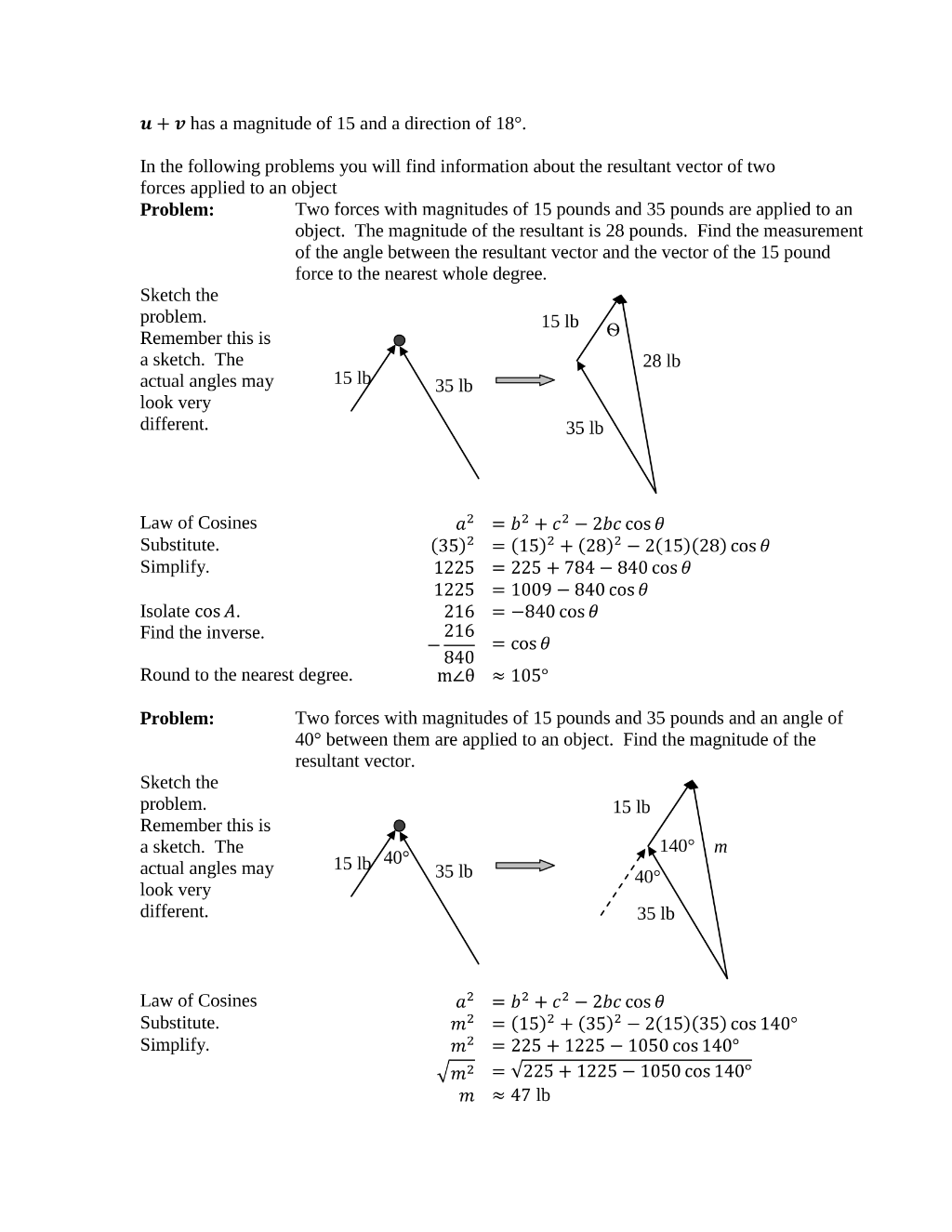
Preview 5
Preview 6